CHAPTER 2 - BASIC CONCEPTS RELATED TO FLOWING WATER AND MEASUREMENT

16. Normal Flow Equations and Friction Head Loss

Many measuring devices, such as flumes, weirs, and submerged orifices, are sensitive to exit flow conditions. Flumes and weirs can be drowned out by too much downstream submergence depth, and submerged orifices can have too little downstream water above the top orifice edge. Therefore, reliable knowledge of exit depth conditions is needed to properly set the elevation of crests and orifices so as to not compromise accuracy. Inaccurate assessment of downstream depth has even made some measuring device installations useless. Good operation and flow depth forecasts are needed to ensure the design effectiveness of new irrigation measurement systems. Designing for the insertion of a new device into an existing system provides a good opportunity to obtain actual field measurements for investigating possible submergence problems.

The use of actual discharge water surface measurements is recommended. In the absence of actual measurements, normal flow equations are often used to predict flow depths.

Normal flow occurs when the water surface slope, S_ws, is the same as the invert or bottom slope, S_o. When normal flow is approached, the velocity equations of Chezy, Manning, and Darcy-Weisbach are used to compute depth of flow. However, these equations are in terms of hydraulic radius, R_h, and depth must be determined on the basis of the definition of R_h, which is the cross-sectional area, A, divided by its wetted perimeter, P_w.

Chezy developed the earliest velocity equation, expressed as:

Manning's equation is more frequently used and is expressed as:

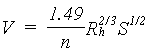
The Darcy-Weisbach equation is a more rigorous relationship, written as:

The coefficients C, n, and f are friction factors. The Darcy-Weisbach friction factor, f, is nondimensional and is a function of Reynolds number, 4R_hV/L, and relative roughness, k/4R_h, in which L is kinematic viscosity, and k is a linear measure of boundary roughness size. The Reynolds number accounts for variation of viscosity. This function is given in the form of plots in any fluid mechanics textbook; for example, Streeter (1951), Rouse (1950), and Chow (1959). These plots are generally in terms of pipe diameter, D, which should be replaced with 4R_h for open channel flow. Values of k have been determined empirically and are constant for a given flow boundary material as long as the roughness can be considered a homogenous texture rather than large roughness elements relative to the depth.

Solving equations 2-51, 2-52, and 2-53 for V/(R_hS)^1/2 results in a combined flow equation and relationship between the three friction factors, C, n, and f, written as:

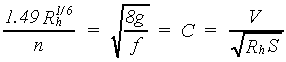
Solving for velocity using equation 2-54 and multiplying by area produces a discharge equation and can be used in the slope area method of determining discharge as discussed in chapter 13.

All three of these friction factors have been determined empirically, computed from measurement of equation variables. The Chezy factor, C, varies with hydraulic radius, slope, and physical boundary roughness. The Chezy factor varies from 22 to 220. Manning's friction factor, n, varies from 0.02 for fine earth lined channels to 0.035 for gravel. If the channel beds are strewn with rocks or are 1/3 full of vegetation, the n value can be as much as 0.06. The n values for concrete vary from 0.011 to 0.016 as finish gets rougher. Values of k can be found in hydraulic and fluid mechanics textbooks such as Streeter (1951), Rouse (1950), and Chow (1959). The value of k for concrete varies from 0.01 to 0.0001 ft depending on condition and quality of finishing. Because Chezy and Manning equations and their friction factors have been determined for ordinary channel flows, they do not accurately apply to shallow flow, nor can these two equations be corrected for temperature viscosity effects. Values of k are constant for given material surfaces for k/4R_h equal or less than 1/10 and when 4R_hV/ is greater than 200,000.

Flow depths downstream are more likely the result of intentional structural restriction or water delivery head requirements downstream. Therefore, in designing and setting the elevation of flumes and weirs, the flow conditions just downstream need to be carefully assessed or specified in terms of required downstream operations and limits of measuring devices. More advanced hydraulic analyses are needed where normal flow is not established. For gradually varied flow, the friction equations can be used as trial and error computations applied to average end section hydraulic variables for relatively short reach lengths. The design and setting of crest elevations in an existing system permit the establishment of operation needs and downstream depths by actual field measurement.