CHAPTER 2 - BASIC CONCEPTS RELATED TO FLOWING WATER AND MEASUREMENT

13. Discharge Equation for Broad-Crested Rectangular Weirs

The discharge equation for the rectangular broad-crested weir will now be derived similar to Bos (1989). The width, L_b, of a rectangular flow section is the same as T, the top water surface width. Also, h_c is the same as h_cm, and using equation 2-29 for velocity head, equation 2-30 can be rewritten as:

or:

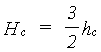
Conversely:

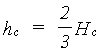
Multiplying both sides of equation 2-27 by the area, A_c, of the flow section, which is L_bh_c, results in discharge expressed as:

To get unit discharge, q, this equation is divided by the width of flow, L_b, resulting in:

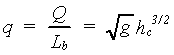
Solving for h_c:

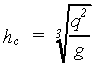
Using equation 2-34 to replace h_c with H_c in equation 2-35 results in theoretical discharge, Q_t:

Discharges in equations 2-35 through 2-38 are usually considered actual, assuming uniform velocity throughout the critical depth cross section and assuming that no correction of velocity distribution is needed.

Because specific energy is constant in a fairly short measuring structure with insignificant friction losses, specific energy, H_c, at the critical location can be replaced with specific energy, H₁, at a head measuring station a short distance upstream. However, some friction loss, possible flow curvature, and nonuniform velocity distribution occur. Thus, a coefficient of C_d must be added to correct for these effects, resulting in an expression for actual discharge:

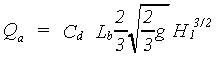
For measurement convenience, the total head, H₁, is replaced with the depth, h₁. To correct for neglecting the velocity head at the measuring station, a velocity coefficient, C_v, must be added, resulting in:

This equation applies to both long-throated flumes or broad-crested weirs and can be modified for any shape by analyses using the energy balance with equation 2-31.

These equations differ only in numerical constants that are derived from assumptions and selection of basic relationships used in their derivation. However, experimental determination of the coefficient values for C and C_v would compensate, making each equation produce the same discharge for the same measuring head. Either equation could be used.

The examples given above show that traditional discharge equations are often a mixture of rational analysis and experimental coefficient evaluation. However, recent development of computer modeling of long-throated flumes (Clemmens et al. [1991]) precludes the need for experimental determination of coefficients. These long-throated flumes are covered in chapter 8.